(2: 中国科学院大学, 北京 100049)
(2: University of Chinese Academy of Sciences, Beijing 100049, P.R. China)
湖泊储存了丰富的水资源,全球共有3.04亿个湖泊(≥0.001 km2),占地面积共计420万km2,占大陆表面积的3% 以上[1]。湖泊参与地球各圈层的相互作用,连接大气圈、水圈和生物圈,在全球水循环系统中发挥着重要的作用。湖泊与陆地相比具有更低的反照率、更大的热容量以及更低的粗糙度,可以吸收更多的太阳辐射,对气温变化起到更大的缓冲作用[2-3]。湖泊与大气之间的水热交换较陆地更为充分,该过程可以改变周围的大气环流,对局地甚至全球范围内的天气和气候状态产生显著影响,并对气候变化产生敏锐的指示作用[4-6]。同时,湖泊蒸发作为湖泊水量平衡中的关键环节,其精确量化可为湖库水量调度和水资源管理提供决策支持,并对区域气候和旱涝变化趋势研究具有重要意义。然而,由于湖泊蒸发观测难度较大,湖泊蒸发过程复杂,使得准确地捕捉并量化湖泊蒸发过程,一直是湖沼学、水文学和气象学等学科的重要研究内容。
早期国内外关于湖泊蒸发的研究多基于蒸发皿观测结果,主要分析水面蒸发的影响要素,并提出各地的蒸发经验公式以及不同蒸发器之间的折算系数。但是传统蒸发皿观测的蒸发量实质上与湖泊实际蒸发量之间存在着明显的差异[7-9],这导致实际的水面蒸发特征仍不清楚,尤其是在小时尺度上。随着技术的进步,波文比仪、涡度相关技术和闪烁仪等高精度仪器已应用于水汽的长期定位观测,为正确认识湖泊实际蒸发过程提供了可能。然而,由于湖泊的形态结构、地理位置和气候背景不同,导致各湖泊在不同时间尺度上的蒸发特征不同,其驱动因素也存在差异[10-11]。理解全球湖泊蒸发机制的共性与差异形成原因对于提出适用性更强的湖泊蒸发计算方法具有重要意义。本文系统梳理了自涡度相关系统等高精度仪器应用以来的国内外湖泊蒸发研究进展,总结了全球湖泊蒸发的实际特征,概述了湖泊蒸发的影响因素,指出了湖泊蒸发研究需要关注的前沿问题。
1 湖泊蒸发测算方法 1.1 观测方法开展湖泊蒸发研究的主要观测方法有蒸发皿法(pan)、波文比法(Bowen ratio)、涡度相关法(eddy covariance system)和闪烁仪法(scintillometer)等[12-13]。
早在14—16世纪的欧洲文艺复兴时期,蒸发皿开始被用于蒸发观测,是最早的蒸发观测仪器。它依据水量平衡原理,根据蒸发皿水位变化得到蒸发量。我国在太湖、宜兴团氿湖、鄱阳湖等地建设过包含100 m2、20 m2或10 m2大型蒸发池的蒸发实验站,并结合E-601型、Φ20 cm小口径、Φ80 cm盆套式的陆地蒸发器和E-601型、гги-3000型的漂浮蒸发器等开展了水面蒸发及其相关水文气象要素的观测研究[14-15]。世界气象组织(WMO)将器口面积为20 m2的蒸发池作为蒸发器的国际参考标准[16]。然而,由于水面和陆面热力、动力条件的差异,陆地蒸发器(皿)观测的蒸发量与湖泊实际蒸发量存在差异,需要进行修正[7, 17]。濮培民提出可用经动力因素修订后的漂浮蒸发器观测的蒸发量作为湖泊实际蒸发量,常用的水面E-601型蒸发器的折算系数为0.89[17]。该方法原理及操作简单,造价低,全球的众多湖泊均有基于此方法的观测数据,积累的观测数据时间序列长,其中中国自20世纪50年代起已有超过60年的观测资料,可用于分析湖泊蒸发长期变化趋势[13]。然而,蒸发皿观测也存在一些局限性,如:测量误差造成的不确定性(飞溅、器壁加热损失、风的影响);冻结期无法使用;储热小;折算系数难以确定等,导致其对于气象过程的响应相比湖泊更加敏感。赵晓松等[8]在鄱阳湖的观测研究中发现蒸发皿蒸发表现为在高值时低估,在低值时高估,更容易受到季节变化的影响。
波文比法以显热通量与潜热通量之比(波文比)概念为基础,依据能量平衡原理,并假设热量和水汽的传输系数相等,通过测量空气的温度梯度和湿度梯度,计算获得地表蒸发量[18],其野外测量系统常称为波文比仪。这一方法的物理原理明确,所需实测参数较少,计算方法简单,观测范围在百米量级。在湖泊水体的蓄热项可以量化的情况下,能够获得较为准确的蒸发量,尤其是小型湖泊[19-20]。该方法要求仪器安装高度有足够的风浪区长度,并通常适用于较长的时间尺度(周、月、年),以减少由于蓄热项、净平流能量项以及其他能量平衡项测量或估算的不确定性及误差[20]。Metzger等[21]在死海的观测研究发现当不考虑蓄热项时,波文比法得到的湖泊潜热通量在春夏季高估,在冬季低估,并造成年蒸发量高估22%。高雅琦等[22]在太湖的研究发现,在考虑了蓄热项后,波文比法与涡度相关法观测值接近,相关系数为0.99,相对误差为-8.6%。
涡度相关法依据涡度协方差理论,通过计算垂直风速和水汽密度脉动值的协方差得到水汽通量,再乘以汽化潜热得到潜热通量(正值为蒸发量,负值为凝结量)。涡度相关法原理严谨,理论假设少,没有经验参数,可以提供潜热通量的直接观测结果,可用于长期定位观测,获得连续的瞬时蒸发量,观测范围在百米量级[18, 23-24]。由于涡度相关仪的传感器制作精密,在野外长期观测需要专业监管,维护保养成本高。当使用该方法时,需要对原始数据进行多项修正,并进行源区分析以获得来自湖面和有代表性的风浪区。涡度相关仪被认为是进行湖泊、水库等内陆水体水热通量观测的最可靠手段[13, 25]。然而,由于局部平流[26]、能量平衡分量源区尺度不匹配[27]以及忽略了低频和高频湍流[28-29],涡度相关法存在能量不闭合问题,造成了潜热通量5%~20% 的误差[27, 30],在水体能量平衡闭合度为70%~97% [31]。
闪烁仪法根据大气中光的闪烁推算大气中的热通量。闪烁仪的发射端发射一定波长的电磁波束,在大气中传播,由接收端接收。电磁波束在传播过程中受到大气温度、湿度和气压波动的影响,根据空气折射系数和莫宁-奥布霍夫相似理论,可计算得到湍流通量。根据光源波长的不同,可分为光学闪烁仪和微波闪烁仪。光学闪烁仪发射信号折射率的变化对温度脉动最为敏感,可以用于测量显热通量,潜热通量是根据能量平衡方程计算得出。微波闪烁仪发射信号折射率的变化对湿度脉动比较敏感,将光学闪烁仪和微波闪烁仪结合起来的双波长闪烁仪可同时测量显热通量和潜热通量。闪烁仪可以在景观尺度上对蒸发进行观测,路径长度为100~1000 m,观测足迹相对稳定,但其理论基础复杂,涉及参数较多[32]。目前在澳大利亚的Logan's Dam[33]和中国的巴丹吉林沙漠湖泊[34]均有利用闪烁仪进行湖泊蒸发的研究。闪烁仪法相比于涡度相关法,观测空间覆盖范围更大,而且受能量平衡不闭合影响较小。McGloin等[33]在澳大利亚Logan's Dam的观测研究发现闪烁仪法测得的潜热通量比涡度相关法观测值大21%。
1.2 数学模型1802年,英国化学家、物理学家Dalton通过实验提出水面蒸发速率与水汽压差(水面温度下的饱和水汽压同水面上空气的实际水汽压的差值)、水面上风速成正比,而与水面上气压成反比,即Dalton蒸发定律[35]。该定律为水面蒸发定量化研究及蒸发模型的发展奠定了理论基础。Dalton定律中包含一个与风速有关的比例系数,其具体数值不易获取,需要通过实验得到,并且对于不同地方的水体没有通用的数值。之后,Dalton指出气压对水面蒸发的影响甚微,可以忽略不计,在Dalton公式中省略了气压项。1882年Stelling提出将Dalton公式中的风速系数替换为风速的一次线性函数,得到了广泛应用[36]。
1915年Schmidt首次利用能量平衡方法得到洋面蒸发[37],但当时该方法中的地表净辐射、显热通量等较难获取,该方法没有得到广泛应用。1926年美国物理学家Bowen从能量平衡角度出发,提出了波文比的概念,用于描述蒸发过程中的能量分配,即波文比能量平衡法(Bowen ratio energy balance, BREB),解决了显热通量难以获取的问题[38]。1939年Thornthwaite和Holzman基于边界层相似理论提出了空气动力学法,通过近地面层的气象要素梯度和湍流扩散系数计算得到潜热通量[39]。1943年水文学家Horton利用水量平衡方程,计算了水库蒸发量[40]。1948年英国物理学家Penman首次将能量平衡法与空气动力学法相结合,提出了Penman公式,将水面蒸发速率分解为由辐射引起的和由湍流输送引起的两项,消除了在当时最难观测的要素——水面温度[41]。该方法物理原理可靠,具有较高的精度,是计算水面蒸发的经典方法。20世纪50—60年代,国外学者提出了多种辐射-温度模型和温度模型,如Makkink模型[42]、Jensen-Haise模型[43]、Hamon模型[44]等。1972年Priestley和Taylor在Penman公式的基础上进行了简化,省去了空气动力学项,在能量项前引入Priestley-Taylor系数(通常取值1.26)[45]。1974年Ryan和Harlenman[46]利用平板传热比拟和天然水温的蒸发公式,将自由对流和强迫对流的作用进行线性叠加,认为强迫对流蒸发和风速线性相关,提出了R-H模型,在欧美得到广泛应用。1979年de Bruin和Keijman在Priestley-Taylor公式的基础上进行了改进,舍去Priestley-Taylor系数,得到了deBruin-Keijman公式[47]。
我国从20世纪50年代开始进行水面蒸发模型研究,主要是根据我国的气候特点和地理条件,对Penman模型、Dalton模型、物质传输模型的参数进行本地化修正。濮培民[9]结合质量守恒原理建立了中国通用的水面蒸发计算公式,称之为中国通用公式A。陈惠泉、毛世民等[48]提出了包含风速和水气温差的水面蒸发计算模型,后称之为中国通用公式B,能够综合反映水面蒸发过程中自由对流和强迫对流的共同作用。李万义[49]提出了包含相对湿度和风速指数函数的中国通用公式C。21世纪国内学者从蒸发物理机理角度入手,提出了考虑辐射、表面温度和蒸发间耦合关系的最大蒸发模型[50]以及基于大气边界层理论的水面蒸发模型[51]。
综上所述,水面蒸发计算模型可分为空气动力学模型(aerodynamics)、能量平衡模型(energy balance)、水量平衡模型(water balance)、综合模型(combination)、辐射-温度模型(solar radiation-temperature)、温度模型(temperature)以及经验公式模型(empirical factors),具体计算公式如表 1所示。
| 表 1 水面蒸发计算模型* Tab. 1 Models for estimating surface water evaporation |
湖泊数量众多,但分布在世界不同的区域。20世纪90年代以来,随着涡度相关系统等观测技术的不断推广普及,湖泊蒸发观测变得可行,相关的研究报道日趋增加[61-62]。根据现有的文献资料,梳理了湖泊蒸发在日内、季节、年际、年代际4种不同时间尺度的变化特征。
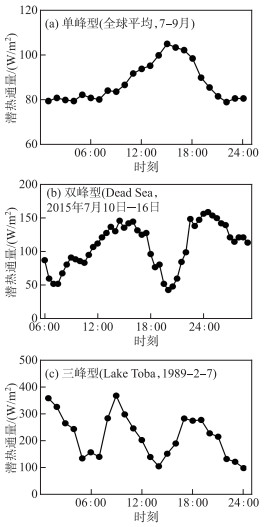
2.1 日内变化特征就全球总体情况而言,湖泊蒸发存在明显的单峰日变化:在15:00左右达到峰值,在傍晚和凌晨时最小(图 1a)[11]。相比陆地蒸发,湖泊蒸发的日内峰值出现较晚:陆面蒸发的峰值通常出现在正午,与净辐射同步;而湖泊蒸发相位迟于陆地,峰值通常出现在午后[63-65]。值得注意的是,少数湖泊的日内蒸发表现为双峰或三峰特征。例如,Lensky等[66]在死海观测到蒸发显示出独特的双峰日变化周期,在下午和夜间均出现了蒸发峰值,分别是由地表净辐射和风速引起的;在日出和日落时分蒸发最小(图 1b)。Sene等[67]在热带湖泊Toba湖观测到3个峰值的蒸发日变化(图 1c),与风速的日变化过程一致。
同一湖泊的日内变化过程也存在着时空异质性。例如,Verburg和Hecky[68]发现不同的风速日内变化特征造成Tanganyika湖南北部蒸发日内变化特征的差异:在湖泊北部,蒸发存在着单峰日变化过程;而在湖泊南部,蒸发却存在双峰日变化过程。Zhao和Liu[69]利用涡度相关系统,在具有水陆季相转换特征的鄱阳湖,观测到在大水面时期,蒸发没有明显的日变化周期;而当洲滩出露,下垫面表现为陆地时,蒸发存在单峰日变化过程。Franz等[70]在西伯利亚北部一个热喀斯特湖的观测发现,在湖泊的冻结、融化和开放水面3个时期,分别存在着不同的蒸发日变化特征:在冻结期,蒸发在夜间较小,在白天为正值,并在15:00达到峰值;在融化期,蒸发全天均为负值,并在17:30左右达到峰值;在开放水面期,蒸发全天为正值,在15:30达到峰值。
陆地的夜间蒸发量通常较小[71-72],而湖泊的夜间蒸发量较大,甚至与日间蒸发量相当。毛锐[7]用100 m2蒸发池在团氿湖的观测发现各月昼夜蒸发量大致相当。Wang等[31]根据涡度相关观测的结果发现中国太湖的夜间蒸发量占全年总蒸发量的48%。在中国的青海湖[73]、鄂陵湖[74]、美国的Ross Barnett水库[75],也有类似的报道。另外,暖季夜间蒸发量往往高于冷季,但冷季夜间蒸发量也仍占较大的比例。Li等[74]在中国鄂陵湖的观测发现,暖季夜间蒸发量比冷季高出约5个百分点。Ross Barnett水库在秋冬季(9—2月)的夜间水分损失占总蒸发水分损失的45% [76]。
总之,湖泊的日内蒸发特征并非单一,不但表现出单峰特征,也可具有双峰、甚至三峰特征。即使在同一湖泊,也可能存在两种以上不同的日变化特征。另外,无论暖季还是冷季,湖泊的夜间蒸发与其日间蒸发量级相当,不可忽视。
2.2 季节变化特征湖泊蒸发量存在显著的单峰季节变化:在暖季增加,冷季减小。然而,月蒸发量达到峰值的时间,浅水湖与深水湖之间差异明显。浅水湖泊蒸发量的峰值主要出现在夏季,呈现夏季高、冬季低的特征[75, 77-78]。例如,亚热带浅水湖泊太湖(平均深度1.9 m),最大蒸发量在7月,最小蒸发量在2月[22]。季节性浅水湖泊鄱阳湖(平均深度8.4 m)蒸发强度在8月最大,1月最小,年内变化过程线呈单峰型[15]。深水湖由于具有更大的储热能力,可以在春夏季储存大量能量并在秋冬季释放,其最大蒸发量主要出现在秋冬季,存在秋冬蒸发显著高于夏季蒸发的现象[79-81],基于浅层蒸发皿的观测往往难以捕捉该现象[13]。例如,北美的Superior湖(平均深度148 m),最大蒸发量在12月,2008—2012年冬季(11月-终冰期)蒸发量是夏季(6—8月)蒸发量的15.2倍[82]。Tasumi[83]总结了日本四个不同深度湖泊的蒸发季节变化。其中,最深的湖泊(Towada湖,平均深度80 m)在夏季(5月)蒸发最小,冬季(11月)蒸发最大;最浅的湖泊(霞浦湖,平均深度3 m)最大蒸发量出现在夏季(8月),而其他两个湖泊的月蒸发量峰值时间介于两者之间(图 2)。因此,湖泊蒸发的季节性变化,也与湖泊深度等形态结构特性之间存在一定的联系。
2.3 年际变化特征蒸发的年际变化在不同湖泊间各异,总的来说,对于高纬度湖泊,蒸发的年际变化幅度相对较大,而对于低纬度湖泊,蒸发的年际变化幅度相对较小。位于高纬度地区的Emaiksoun湖(71.23°N)、Great Slave湖(61.60°N)湖泊蒸发年际变化的变差系数分别为0.11(3年)[84]、0.12(3年)[81]。在中纬度地区,Perch湖(46.03°N)年平均蒸发量的11年变差系数为0.08[85],Mirror湖(43.62°N)年平均蒸发量的6年变差系数为0.09[20],位于较低纬度的Ross Barnett水库(32.43°N)、太湖(31.25°N)蒸发年变差系数相对较小,分别为0.05(2年)[86]、0.05(7年)[87],其中太湖潜热通量在2010—2017年间呈增加趋势,增加率为(1.2±1.9) W/(m2 ·a)。位于低纬度地区的Nasser湖(22.98°N)在1995—2004年间没有明显的增加或减小趋势,蒸发年变差系数为0.03[88],低于中高纬度地区的湖泊。因此,湖泊蒸发的年际变化幅度与所处纬度存在一定关联。另外,对于中高纬度地区的冰冻湖泊,蒸发年际间的差异与冰层面积、冰期持续时间有关,冰层面积大、封冻期长的年份湖泊蒸发量小。Blanken等[25]在Superior湖的观测研究发现,具有较大冰覆盖面积的2008—2009年(最大90%)比冰覆盖面积较小的2009—2010年(最大31%)蒸发量小28%。
2.4 年代际变化趋势基于模型模拟的结果显示,全球湖泊/水库及其周边地区的蒸发量在过去几十年有所增加[89]。对于单个湖泊,荆思佳等[90]利用CLM4-LISSS模型对太湖的蒸发量进行了模拟,结果表明1958—2017年湖泊蒸发量呈增加趋势,增加速率为1.4 mm/a(图 3a)。Xiao等[91]基于CLM4-LISSS模型的模拟结果显示White Bear湖年蒸发量在1979—2016年以3.8 mm/a的速度增加(图 3b)。Karaoun湖的年蒸发量在1995—2018年间呈增加趋势(图 3c),其中年平均蒸发速率在1985—1989年(1.87 mm/d)和2014—2018年(3.12 mm/d)间几乎翻了一番[92]。Wang等[89]利用数值模型,对未来情景进行模拟,发现未来湖泊蒸发仍存在增加趋势,其中,2006—2100年全球湖泊蒸发的平均增加量为140 mm (11 W/m2),增长了16%。低纬度地区(30°S~30°N)的增加量更明显,为210 mm (16.5 W/m2)。Mhawej等[92]通过模拟发现Karaoun湖的年蒸发量从2018年到2038年将增加55%,而到2068年将增加100%。然而,基于蒸发皿观测的结果显示一些湖泊的蒸发年代际变化呈现出减小趋势。例如,闵骞等[93]分析了鄱阳湖1955—2004年水面蒸发的变化趋势,得出鄱阳湖年蒸发量呈递减趋势,平均每年减少2.79 mm(图 3d)。时兴合等[94]利用蒸发皿观测资料,发现1958—2007年青海湖年蒸发量逐步减小,总减小量为120 mm(图 3e)。1962—2008年洱海蒸发皿的观测结果显示,由于云量增多,水面蒸发量呈减小趋势,总减小量为128 mm(图 3f)[95]。由此可见,关于湖泊蒸发的多年变化趋势仍需进一步验证。
3 湖泊蒸发的影响因素湖泊蒸发特征的形成离不开影响蒸发的各种驱动因素。从湖-气相互作用的界面过程来看,水热交换受水汽压差、风、辐射等要素的影响。从大尺度时空过程来看,湖泊蒸发也与其结构形态(表面面积、深度等)和所处的地理位置(纬度、海拔等)等因素之间存在必然的联系。
3.1 不同时间尺度湖泊蒸发的影响因素湖-气界面水热交换受水汽压差、风、辐射等要素的影响,然而,在不同时间尺度上,影响湖泊蒸发的主要因素并不相同。
在小时至日尺度上,湖泊蒸发受风速和水气界面水汽压差的协同作用影响[76, 96],符合Dalton系列模型的理论。水面的饱和水汽压大于水面上方空气中的水汽压,引起水汽由水面向上方空气中扩散,近水体表层空气中的水汽压下降,为了使水体表面上水汽趋于饱和,就会有新的水分子汽化逸出水面进入上方空气中,形成了水面蒸发[97]。因此,水面蒸发与水汽压差呈正比,当水汽压差越大时,水分子越容易逸出水面,蒸发量越大。风通过加剧湍流扩散作用,带走水面上方的水汽而使其不易达到饱和,促进水汽交换,进而间接影响蒸发。湖泊蒸发在日内尺度上对风的响应较为迅速,风速的瞬时增加会导致水体蒸发率骤然上升,造成湖泊蒸发出现双峰或多峰的日内变化特征。
在月尺度和季节变化上,湖泊蒸发主要受地表净辐射的影响[19, 98],地表净辐射是地球表面短波与长波辐射平衡而产生的净能量,是蒸发的主要能量来源。然而,由于水体储热的缓冲作用,造成潜热通量年内峰值往往滞后于地表净辐射,在浅水湖滞后时间通常为1个月[31, 77, 86],而在深水湖滞后时间可长达5个月[25, 99-100]。在消除滞后影响后,月均潜热通量与净辐射量之间存在明显的相关关系[101]。
季节内湖泊蒸发的变化主要与天气的变化有关,高蒸发量事件通常发生在干冷气团入侵期间。天气冷锋与冷锋后长时间的干冷气团,通过增强大气湍流的热和动力混合,显著地促进湍流交换,从而引起潜热产生短期的快速脉冲损失,增大蒸发量[19, 102]。Liu等[76]发现冷锋过境期间,日蒸发量为非事件日的7.3倍,使得Ross Barnett水库秋冬季的蒸发量增加了157%。Li等[73]也指出,青海湖的蒸发会受到冷锋与干冷气团侵入引起的大风事件的频率、强度和持续时间等因素的控制。相较于深水湖泊,浅水湖泊对于冷空气事件的响应更加迅速。在中国的太湖(平均深度1.9 m),寒潮、强冷空气和较强冷空气过境使得潜热通量比平时分别增加了4.0、2.1、2.7倍[103]。
湖泊蒸发的年际变化和年代际变化主要受湖泊能量输入的影响。Xiao等[87]基于涡度相关系统在太湖7年的观测研究表明,入射短波辐射和入射长波辐射的增加是湖泊蒸发增强的主要原因,而地表反馈的长波辐射则会减弱湖泊蒸发。Fink等[104]、Hu等[105]、Lazhu等[100]分别在Constance湖、太湖、纳木错的研究中指出,在年代际上湖泊蒸发的增加是由于入射辐射的增加。在气候变暖背景下,对于热带和温度湖泊,气温升高引起波文比减小,从而改变了地表可利用能量的分配比例,更多的能量用于蒸发,湖泊蒸发增加;对于寒带和极地的结冰湖面,温度升高造成结冰期缩短,湖面反照率下降,更多的能量被湖体吸收,进而促进蒸发[89]。故在年代际变化上,由于能量输入的增加,湖泊蒸发呈增加趋势。对于开阔湖面,湖面温度上升速率慢于气温,上行长波辐射减小,更多的能量用于蒸发,放大了湖泊蒸发对变暖的响应;对于结冰湖泊,湖面温度与气温的上升速率相当,上行长波辐射增加,用于蒸发的能量变少,产生负反馈,放缓对变暖的响应[89]。因此,在增温情境下,开阔湖面湖泊蒸发增加量更大。
3.2 湖泊形态结构对湖泊蒸发的影响湖泊表面面积等形态结构特性会影响湖泊水体及其与大气边界层之间的相互作用。湖泊面积会影响风速进而影响蒸发量,它与风速之间存在明显的正相关关系,但不影响水汽压差[11]。大型湖泊由于风速大、风浪大,强风和湖体热对流混合了上层湖水,将湖体储存的热量带到表面,之后强烈的动力湍流和自由对流将其输送到大气中,使得蒸发增加。小湖泊受到平流影响,也会促进蒸发[64, 106]。湖泊面积也影响湖面温度,湖面温度日变化幅度随湖泊面积的增加而下降[107]。当湖面温度超过空气温度时,会产生不稳定的大气边界层,湖面温度相对于气温越高,大气边界层越不稳定,因潜热和显热引起的湖泊热量损失也就越多[52]。季节性湖泊水相陆相的交替,高纬度或高海拔湖泊冻结期与融化期的转换,以及湖泊的萎缩与扩展,都会引起湖泊表面面积的变化,从而影响湖泊蒸发。
湖泊深度对湖泊蒸发的影响主要是通过改变水体储热量来调节能量分布,进而影响湖泊热量收支,改变湖泊蒸发及其变化规律[108]。由于湖泊相较陆地反照率更低、比热容更大、热传导率更低,使其具有较大的储热能力,湖泊水体所吸收太阳辐射的一部分,将用于加热水体并储存起来,导致蒸发峰值在季节尺度上滞后于辐射峰值。蒸发峰值迟于净辐射的滞后时间,与湖泊水深之间存在显著的相关关系[96]:湖泊越深,储热能力越强,具有更大的热惯量,滞后时间越长,蒸发的年内峰值出现的越晚;当深度达到一定的临界值后,滞后时间不再增加。因此,相较于浅水湖泊,深水湖泊蒸发对于能量变化的响应通常需要更长的时间。Wang等[80]分析了纳木错及其邻近小湖的湖-气相互作用差异。较深的纳木错具有更大的储热能力,使其结冰期和融化期相对延后,水面温度、气温、潜热和显热的季节峰值也相对延后。尽管湖泊深度对湖泊蒸发的影响显著,但目前湖泊模型还难以充分地刻画湖泊热惯量的作用,有待加强这方面的研究[109]。
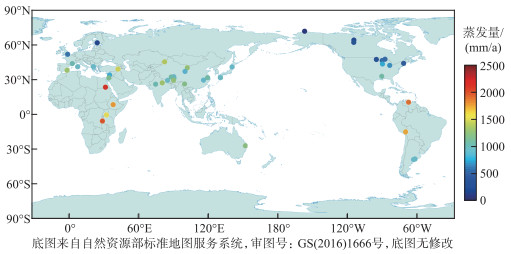
3.3 地理因素对湖泊蒸发的影响纬度和海拔等地理因素通过气象要素变化而影响湖泊蒸发量及其变化规律。不同纬度的太阳高度角不同,各湖泊接收到的太阳辐射量和气温等要素大小各异。气温通过影响波文比,改变地表可利用能量的分配比例,进而影响蒸发。随着纬度的降低,波文比随气温上升而下降,分配给蒸发的能量增加,潜热对总湍流热损失的相对贡献增加。Woolway等[11]分析了全球45个湖泊的湍流通量特征,总结得出:在低纬度热带地区,潜热通量对湍流热损失的贡献可超过90%;而随着纬度的升高,潜热通量对湍流热损失的贡献减小,在高纬度地区只有约60%。图 4展示了全球不同地区典型湖泊的年均蒸发量(站点信息见附表Ⅰ),图 5给出了湖泊年蒸发量与湖泊所处纬度、海拔之间的关系。由图 4、图 5可知,湖泊年蒸发量与纬度间存在明显的负相关关系(R2=0.69):湖泊蒸发随纬度的增加而减小。低(0°~30°)、中(30°~60°)、高(60°~90°)纬度地区的平均湖泊年蒸发量分别为1459.6、953.4、252.6 mm。纬度不仅影响潜热通量的分配比例,同时影响潜热通量的季节变化[11]。随着纬度的升高,热收支的变化幅度增加。低纬度湖泊潜热通量的季节变化不明显,月均值近似恒定;而中高纬度湖泊的季节变化更为明显。在增温情景下,低纬度湖泊蒸发将比中高纬度湖泊具有更高的年际增长率[89]。
|
图 4 全球湖泊蒸发观测站点及湖泊年蒸发量 Fig.4 Global distribution of lake observation sites and annual mean lake evaporation |

|
图 5 湖泊年蒸发量与湖泊所处纬度、海拔之间的关系 Fig.5 Relationship of annual mean lake evaporation with latitude and altitude |
海拔对蒸发的影响主要是通过间接作用产生的。Woolway等[11]利用统计方法分析得出海拔与湖泊蒸发间没有明显的关联。从图 5中也可以看出,湖泊年蒸发量与海拔间没有明显的相关关系。相较于海拔因素,温度与湖泊蒸发之间的关系更为密切,由海拔升高而引起的温度减小对蒸发的影响更大[110]。另外,大尺度大气环流引发的逆温(气温随海拔的增加而增加),也会使得在一定高度段蒸发随海拔的升高而增加[111]。总之,纬度和海拔等地理因素是通过影响气象要素而间接地影响湖泊蒸发。
4 结论与展望水面蒸发研究已具有上百年的历史,但直到20世纪90年代,随着涡度相关系统观测技术的不断推广普及,湖泊的实际蒸发观测才变得可行。由于具有更低的反照率、更大的热容量以及更低的粗糙度,湖泊的蒸发呈现出与陆地不同的变化特征。在日内过程上,湖泊蒸发可表现为单峰、双峰、甚至三峰,夜间蒸发量略低于日间蒸发量,但量级相当;在季节上,湖泊蒸发表现出单峰特征,但浅水湖与深水湖不同,浅水湖夏季高冬季低,而深水湖秋冬高;在年际上,高纬度湖泊比低纬度湖泊蒸发年际变化幅度大;在年代际上,过去数十年来全球湖泊蒸发总体呈增加趋势。
在影响要素上,湖泊蒸发受到水汽压差、风、辐射等要素的作用,不同时间尺度上的主控因素也不尽相同。在小时至日尺度上,受风速和水汽压差的协同控制;在月和季节尺度上,净辐射影响变得更为突出;在季节内,干冷气团入侵会引起蒸发脉冲事件;在年际和年代际尺度上,受湖泊能量输入的影响。除气象要素外,湖泊蒸发还与湖泊面积和深度等形态结构及纬度和海拔等地理因素有关。湖泊面积通过影响风速而影响蒸发量;湖泊深度通过影响水体储热量而影响蒸发的季节变化,深水湖蒸发的年内峰值通常出现较晚。纬度通过改变气温和净辐射而影响湖泊蒸发量及季节变幅,湖泊蒸发随纬度的升高而减小;海拔则通过改变气温等因素,间接影响湖泊蒸发。
全球湖泊分布较为分散,湖泊之间的蒸发差异明显,风浪等因素导致湖泊观测难度大且造价高,内陆水体的实际蒸发观测发展相对滞后,仍有许多问题亟待解决。首先,在观测手段上,即使是较为可靠的涡度相关法,也存在难以避免的能量不闭合问题。其次,在过程研究上,湖泊蒸发不是一个单一的液-汽相变过程,而是受诸多因素的叠加影响,并存在时间尺度相关的反馈以及空间异质性,有关湖泊蒸发的过程机理,还需要进一步深入研究。
5 附录附表Ⅰ见电子版(DOI: 10.18307/2023.0501)。
| 附表Ⅰ 全球湖泊蒸发观测站点信息及湖泊年蒸发量* Appendix Ⅰ Global distribution of lake observation sites and annual mean lake evaporation |
| [1] |
Downing JA, Prairie YT, Cole JJ et al. The global abundance and size distribution of lakes, ponds, and impoundments. Limnology and Oceanography, 2006, 51(5): 2388-2397. DOI:10.4319/lo.2006.51.5.2388 |
| [2] |
Long Z, Perrie W, Gyakum J et al. Northern lake impacts on local seasonal climate. Journal of Hydrometeorology, 2007, 8(4): 881-896. DOI:10.1175/jhm591.1 |
| [3] |
Nordbo A, Launiainen S, Mammarella I et al. Long-term energy flux measurements and energy balance over a small boreal lake using eddy covariance technique. Journal of Geophysical Research: Atmospheres, 2011, 116(D2): D02119. DOI:10.1029/2010jd014542 |
| [4] |
Adrian R, O'Reilly CM, Zagarese H et al. Lakes as sentinels of climate change. Limnology and Oceanography, 2009, 54(6part2): 2283-2297. DOI:10.4319/lo.2009.54.6_part_2.2283 |
| [5] |
Dutra E, Stepanenko VM, Balsamo G et al. An offline study of the impact of lakes on the performance of the ECMWF surface scheme. Boreal Environment Research, 2010, 15(2): 100-112. |
| [6] |
Tornblom K, Bergstrom H, Johansson C. Thermally driven mesoscale flows-simulations and measurements. Boreal Environment Research, 2007, 12(6): 623-641. |
| [7] |
Mao R. Preliminary experimental study of evaporation from water surface of Lake Tai and Lake Tuangui, Jiangsu province. Oceanologia et Limnologia Sinica, 1978, 9(1): 26-35. [毛锐. 太湖、团氿湖水面蒸发的初步研究. 海洋与湖沼, 1978, 9(1): 26-35.] |
| [8] |
Zhao XS, Li M, Wang SG et al. Comparison of actual water evaporation and pan evaporation in summer over the Lake Poyang, China. J Lake Sci, 2015, 27(2): 343-351. [赵晓松, 李梅, 王仕刚等. 鄱阳湖夏季水面蒸发与蒸发皿蒸发的比较. 湖泊科学, 2015, 27(2): 343-351. DOI:10.18307/2015.0220] |
| [9] |
Pu PM. Studies on the formulae for calculating evaporation and heat loss coefficient from water surface in China (Ⅰ). J Lake Sci, 1994, 6(1): 1-12. [濮培民. 水面蒸发与散热系数公式研究(一). 湖泊科学, 1994, 6(1): 1-12. DOI:10.18307/1994.0101] |
| [10] |
Du Q, Liu H, Liu Y et al. Factors controlling evaporation and the CO2 flux over an open water lake in southwest of China on multiple temporal scales. International Journal of Climatology, 2018, 38(13): 4723-4739. DOI:10.1002/joc.5692 |
| [11] |
Woolway RI, Verburg P, Lenters JD et al. Geographic and temporal variations in turbulent heat loss from lakes: A global analysis across 45 lakes. Limnology and Oceanography, 2018, 63(6): 2436-2449. DOI:10.1002/lno.10950 |
| [12] |
Liu YB, Qiu GY, Zhang HS et al. Shifting from homogeneous to heterogeneous surfaces in estimating terrestrial evapotranspration: Review and perspectives. Science China Earth Sciences, 2022, 52(2): 197-214. [刘元波, 邱国玉, 张宏昇等. 陆域蒸散的测算理论方法: 回顾与展望. 中国科学: 地球科学, 2022, 52(3): 381-399. DOI:10.1007/s11430-020-9834-y] |
| [13] |
Friedrich K, Grossman RL, Huntington J et al. Reservoir evaporation in the Western United States: Current science, challenges, and future needs. Bulletin of the American Meteorological Society, 2018, 99(1): 167-187. DOI:10.1175/BAMS-D-15-00224.1 |
| [14] |
毛锐, 高俊峰. 太湖地区湖泊水面蒸发. 北京: 科学技术文献出版社, 1994.
|
| [15] |
Min Q. Preliminary study on water evaporation of Poyang Lake. Hydrology, 1994(6): 35-42. [闵骞. 鄱阳湖水面蒸发规律初探. 水文, 1994(6): 35-42.] |
| [16] |
World Meteorological Organization (WMO). Guide to Meteorolgical Instruments and Methods of Oberservation. WMO-No. 8, 6th Editon. Geneva: World Meteorological Organization, 1996.
|
| [17] |
濮培民. 影响水面蒸发诸因素与蒸发折算系数. 中国科学院南京地理研究所集刊, 1984, 2(2): 1-13. |
| [18] |
Wang K, Dickinson RE. A review of global terrestrial evapotranspiration: Observation, modeling, climatology, and climatic variability. Reviews of Geophysics, 2012, 50(2): RG2005. DOI:10.1029/2011rg000373 |
| [19] |
Lenters JD, Kratz TK, Bowser CJ. Effects of climate variability on lake evaporation: Results from a long-term energy budget study of Sparkling Lake, northern Wisconsin (USA). Journal of Hydrology, 2005, 308(1): 168-195. DOI:10.1016/j.jhydrol.2004.10.028 |
| [20] |
Winter TC, Buso DC, Rosenberry DO et al. Evaporation determined by the energy-budget method for Mirror Lake, New Hampshire. Limnology and Oceanography, 2003, 48(3): 995-1009. DOI:10.4319/lo.2003.48.3.0995 |
| [21] |
Metzger J, Nied M, Corsmeier U et al. Dead Sea evaporation by eddy covariance measurements vs. aerodynamic, energy budget, Priestley-Taylor, and Penman estimates. Hydrology and Earth System Sciences, 2018, 22(2): 1135-1155. DOI:10.5194/hess-22-1135-2018 |
| [22] |
Gao YQ, Wang YW, Hu C et al. Variability of evaporation from Lake Taihu in 2012 and evaluation of a range of evaporation models. Climatic and Environmental Research, 2016, 21(4): 393-404. [高雅琦, 王咏薇, 胡诚等. 2012年太湖蒸发量变化特征及蒸发模型评估研究. 气候与环境研究, 2016, 21(4): 393-404. DOI:10.3878/j.issn.1006-9585.2015.15090] |
| [23] |
Aubinet M, Grelle A, Ibrom A et al. Estimates of the annual net carbon and water exchange of forests: The EUROFLUX methodology. Advances in Ecological Research, 1999, 30: 113-175. DOI:10.1016/S0065-2504(08)60018-5 |
| [24] |
Baldocchi D, Falge E, Gu L et al. FLUXNET: A new tool to study the temporal and spatial variability of ecosystem-scale carbon dioxide, water vapor, and energy flux densities. Bulletin of the American Meteorological Society, 2001, 82(11): 2415-2434. DOI:10.1175/1520-0477(2001)082<2415:FANTTS>2.3.CO;2 |
| [25] |
Blanken PD, Spence C, Hedstrom N et al. Evaporation from Lake Superior: 1. Physical controls and processes. Journal of Great Lakes Research, 2011, 37(4): 707-716. DOI:10.1016/j.jglr.2011.08.009 |
| [26] |
Oncley SP, Foken T, Vogt R et al. The energy balance experiment EBEX-2000. Part Ⅰ: Overview and energy balance. Boundary-Layer Meteorology, 2007, 123(1): 1-28. DOI:10.1007/s10546-007-9161-1 |
| [27] |
Foken T. The energy balance closure problem: An overview. Ecological Applications, 2008, 18(6): 1351-1367. DOI:10.1890/06-0922.1 |
| [28] |
Majozi NP, Mannaerts CM, Ramoelo A et al. Analysing surface energy balance closure and partitioning over a semi-arid savanna FLUXNET site in Skukuza, Kruger National Park, South Africa. Hydrology and Earth System Sciences, 2017, 21(7): 3401-3415. DOI:10.5194/hess-21-3401-2017 |
| [29] |
Sakai RK, Fitzjarrald DR, Moore KE. Importance of low-frequency contributions to eddy fluxes observed over rough surfaces. Journal of Applied Meteorology, 2001, 40(12): 2178-2192. DOI:10.1175/1520-0450(2001)040<2178:iolfct>2.0.co;2 |
| [30] |
Vickers D, Göckede M, Law B. Uncertainty estimates for 1-h averaged turbulence fluxes of carbon dioxide, latent heat and sensible heat. Tellus B: Chemical and Physical Meteorology, 2010, 62(2): 87-99. DOI:10.1111/j.1600-0889.2009.00449.x |
| [31] |
Wang W, Xiao W, Cao C et al. Temporal and spatial variations in radiation and energy balance across a large freshwater lake in China. Journal of Hydrology, 2014, 511: 811-824. DOI:10.1016/j.jhydrol.2014.02.012 |
| [32] |
Moene AF, Beyrich F, Hartogensis OK. Developments in Scintillometry. Bulletin of the American Meteorological Society, 2009, 90(5): 694-698. DOI:10.1175/2008bams2672.1 |
| [33] |
McGloin R, McGowan H, McJannet D et al. Quantification of surface energy fluxes from a small water body using scintillometry and eddy covariance. Water Resources Research, 2014, 50(1): 494-513. DOI:10.1002/2013WR013899 |
| [34] |
Han P, Wang X, Wang J. Using large-aperture scintillometer to estimate lake-water evaporation and heat fluxes in the Badain Jaran Desert, China. Water, 2019, 11(12): 2575. DOI:10.3390/w11122575 |
| [35] |
Dalton J. Experimental essays on the constitution of mixed gases. Manchester Literary and Philosophical Society Memo, 1802, 5: 535-602. |
| [36] |
Brutsaert W. Evaporation into the atmosphere: Theory, history and applications. Dordrecht: Kluwer Academic Publishers, 1982.
|
| [37] |
Schmidt W. Strahlung und Verdunstung an freien Wasserflächen: ein Beitrag zum Wärmehaushalt des Weltmeers und zum Wasserhaushalt der Erde. Ann Calender Hydrographie und Maritimen Meteorologie, 1915, 43: 111-124. |
| [38] |
Bowen IS. The ratio of heat losses by conduction and by evaporation from any water surface. Physical Review, 1926, 27(6): 779-787. DOI:10.1103/PhysRev.27.779 |
| [39] |
Thornthwaite C, Holzman B. The determination of evaporation from land and water surfaces. Monthly Weather Review, 1939, 67(1): 4-11. DOI:10.1175/1520-0493(1939)67<4:TDOEFL>2.0.CO;2 |
| [40] |
Horton RE. Hydrologic interrelations between lands and oceans. Eos, Transactions American Geophysical Union, 1943, 24(2): 753-764. DOI:10.1029/TR024i002p00753 |
| [41] |
Penman HL. Natural evaporation from open water, bare soil and grass. Proceedings of the Royal Society of London. Series A. Mathematical and Physical Sciences, 1948, 193(1032): 120-145. DOI:10.1098/rspa.1948.0037 |
| [42] |
Makkink G. Testing the Penman formula by means of lysimeters. Journal of the Institution of Water Engineerrs, 1957, 11: 277-288. |
| [43] |
Jensen ME, Haise HR. Estimating evapotranspiration from solar radiation. Journal of the Irrigation and Drainage Division, 1963, 89(4): 15-41. DOI:10.1061/JRCEA4.0000287 |
| [44] |
Hamon WR. Estimating potential evapotranspiration. Journal of the Hydraulics Division, 1961, 87(3): 107-120. DOI:10.1061/JYCEAJ.0000599 |
| [45] |
Priestley CHB, Taylor RJ. On the assessment of surface heat flux and evaporation using large-scale parameters. Monthly Weather Review, 1972, 100(2): 81-92. DOI:10.1175/1520-0493(1972)100<0081:otaosh>2.3.co;2 |
| [46] |
Ryan PJ, Harleman DRF, Stolzenbach KD. Surface heat loss from cooling ponds. Water Resources Research, 1974, 10(5): 930-938. DOI:10.1029/WR010i005p00930 |
| [47] |
de Bruin HAR, Keijman JQ. The Priestley-Taylor evaporation model applied to a large, shallow lake in the Netherlands. Journal of Applied Meteorology, 1979, 18(7): 898-903. DOI:10.1175/1520-0450(1979)018<0898:TPTEMA>2.0.CO;2 |
| [48] |
Chen HQ, He SC, Liu CG et al. Experimental study on mass and heat exchange at air-water interface of heated water body. Journal of Hydraulic Engineering, 1989(10): 27-36. [陈惠泉, 何树椿, 刘长贵等. 超温水体水面蒸发与散热. 水利学报, 1989(10): 27-36.] |
| [49] |
Li WY. A Study on the generalized model of water surface evaporation. Hydrology, 2000, 20(4): 13-17. [李万义. 适用于全国范围的水面蒸发量计算模型的研究. 水文, 2000, 20(4): 13-17.] |
| [50] |
Yang Y, Roderick ML. Radiation, surface temperature and evaporation over wet surfaces. Quarterly Journal of the Royal Meteorological Society, 2019, 145(720): 1118-1129. DOI:10.1002/qj.3481 |
| [51] |
Liu Z, Yang H. Estimation of water surface energy partitioning with a conceptual atmospheric boundary layer model. Geophysical Research Letters, 2021, 48(9): e2021GL092643. DOI:10.1029/2021GL092643 |
| [52] |
Garratt JR. Review: The atmospheric boundary layer. Earth-Science Reviews, 1994, 37(1): 89-134. DOI:10.1016/0012-8252(94)90026-4 |
| [53] |
Liu WT, Katsaros KB, Businger JA. Bulk parameterization of air-sea exchanges of heat and water vapor including the moledular constraints at the interface. Journal of Atmospheric Sciences, 1979, 36(9): 1722-1735. |
| [54] |
Stannard DI, Rosenberry DO. A comparison of short-term measurements of lake evaporation using eddy correlation and energy budget methods. Journal of Hydrology, 1991, 122(1): 15-22. DOI:10.1016/0022-1694(91)90168-H |
| [55] |
de Bruin HAR. A simple model for shallow lake evaporation. Journal of Applied Meteorology, 1978, 17(8): 1132-1134. |
| [56] |
Turc L. Estimation of irrigation water requirements, potential evapotranspiration: A simple climatic formula evolved up to date. Annals of Agronomy, 1961, 12(1): 13-49. |
| [57] |
Stephens JC, Stewart EH. A comparison of procedures for computing evaporation and evapotranspiration. Publication, 1963, 62: 123-133. |
| [58] |
Hargreaves GH. Moisture availability and crop production. Transactions of the ASAE, 1975, 18(5): 980-0984. DOI:10.13031/2013.36722 |
| [59] |
Blaney HF, Criddle WD. Determining consumptive use and irrigation water requirements. US Department of Agriculture, 1962.
|
| [60] |
Papadakis J. Potential evapotranspiration. Soil Science, 1965, 100(1): 76. |
| [61] |
Blanken PD, Rouse WR, Culf AD et al. Eddy covariance measurements of evaporation from Great Slave Lake, Northwest Territories, Canada. Water Resources Research, 2000, 36(4): 1069-1077. DOI:10.1029/1999WR900338 |
| [62] |
Hamdani I, Assouline S, Tanny J et al. Seasonal and diurnal evaporation from a deep hypersaline lake: The Dead Sea as a case study. Journal of Hydrology, 2018, 562: 155-167. DOI:10.1016/j.jhydrol.2018.04.057 |
| [63] |
Cui Y, Liu Y, Gan G et al. Hysteresis behavior of surface water fluxes in a hydrologic transition of an ephemeral lake. Journal of Geophysical Research: Atmospheres, 2020, 125(12): e2019JD032364. DOI:10.1029/2019JD032364 |
| [64] |
Assouline S, Tyler SW, Tanny J et al. Evaporation from three water bodies of different sizes and climates: Measurements and scaling analysis. Advances in Water Resources, 2008, 31(1): 160-172. DOI:10.1016/j.advwatres.2007.07.003 |
| [65] |
Venäläinen A, Frech M, Heikinheimo M et al. Comparison of latent and sensible heat fluxes over boreal lakes with concurrent fluxes over a forest: Implications for regional averaging. Agricultural and Forest Meteorology, 1999, 98-99: 535-546. DOI:10.1016/S0168-1923(99)00100-8 |
| [66] |
Lensky NG, Lensky IM, Peretz A et al. Diurnal course of evaporation from the Dead Sea in summer: A distinct double peak induced by solar radiation and night sea breeze. Water Resources Research, 2018, 54(1): 150-160. DOI:10.1002/2017WR021536 |
| [67] |
Sene KJ, Gash JHC, McNeil DD. Evaporation from a tropical lake: Comparison of theory with direct measurements. Journal of Hydrology, 1991, 127(1): 193-217. DOI:10.1016/0022-1694(91)90115-X |
| [68] |
Verburg P, Hecky RE. Wind patterns, evaporation, and related physical variables in Lake Tanganyika, East Africa. Journal of Great Lakes Research, 2003, 29(S2): 48-61. DOI:10.1016/S0380-1330(03)70538-3 |
| [69] |
Zhao X, Liu Y. Phase transition of surface energy exchange in China's largest freshwater lake. Agricultural and Forest Meteorology, 2017, 244: 98-110. DOI:10.1016/j.agrformet.2017.05.024 |
| [70] |
Franz D, Mammarella I, Boike J et al. Lake-atmosphere heat flux dynamics of a thermokarst lake in Arctic Siberia. Journal of Geophysical Research: Atmospheres, 2018, 123(10): 5222-5239. DOI:10.1029/2017JD027751 |
| [71] |
Brutsaert W. Hydrology: An introduction. Cambridge: Cambridge University Press, 2005.
|
| [72] |
Launiainen S. Seasonal and inter-annual variability of energy exchange above a boreal Scots pine forest. Biogeosciences, 2010, 7(12): 3921-3940. DOI:10.5194/bg-7-3921-2010 |
| [73] |
Li X, Ma Y, Huang Y et al. Evaporation and surface energy budget over the largest high-altitude saline lake on the Qinghai-Tibet Plateau. Journal of Geophysical Research: Atmospheres, 2016, 121(18): 10470-10485. DOI:10.1002/2016jd025027 |
| [74] |
Li Z, Lyu S, Ao Y et al. Long-term energy flux and radiation balance observations over Lake Ngoring, Tibetan Plateau. Atmospheric Research, 2015, 155: 13-25. DOI:10.1016/j.atmosres.2014.11.019 |
| [75] |
Liu H, Zhang Q, Dowler G. Environmental controls on the surface energy budget over a large southern inland water in the United States: An analysis of one-year eddy covariance flux data. Journal of Hydrometeorology, 2012, 13(6): 1893-1910. DOI:10.1175/jhm-d-12-020.1 |
| [76] |
Liu H, Zhang Y, Liu S et al. Eddy covariance measurements of surface energy budget and evaporation in a cool season over southern open water in Mississippi. Journal of Geophysical Research: Atmospheres, 2009, 114(D4): D04110. DOI:10.1029/2008JD010891 |
| [77] |
Cui Y, Zhang X, Liu Y. Radiative and aerodynamic contribution to evaporation: Eddy-covariance comparison between a plain and a plateau lake. Earth and Space Science, 2021, 8(10): e2021EA001913. DOI:10.1029/2021EA001913 |
| [78] |
Mammarella I, Nordbo A, Rannik U et al. Carbon dioxide and energy fluxes over a small boreal lake in Southern Finland. Journal of Geophysical Research: Biogeosciences, 2015, 120(7): 1296-1314. DOI:10.1002/2014jg002873 |
| [79] |
Momii K, Ito Y. Heat budget estimates for Lake Ikeda, Japan. Journal of Hydrology, 2008, 361(3): 362-370. DOI:10.1016/j.jhydrol.2008.08.004 |
| [80] |
Wang B, Ma Y, Wang Y et al. Significant differences exist in lake-atmosphere interactions and the evaporation rates of high-elevation small and large lakes. Journal of Hydrology, 2019, 573: 220-234. DOI:10.1016/j.jhydrol.2019.03.066 |
| [81] |
Rouse WR, Oswald CM, Binyamin J et al. Interannual and seasonal variability of the surface energy balance and temperature of central Great Slave Lake. Journal of Hydrometeorology, 2003, 4(4): 720-730. |
| [82] |
Spence C, Blanken PD, Lenters JD et al. The importance of spring and autumn atmospheric conditions for the evaporation regime of lake superior. Journal of Hydrometeorology, 2013, 14(5): 1647-1658. DOI:10.1175/JHM-D-12-0170.1 |
| [83] |
Tasumi M. A review of evaporation research on Japanese lakes. World Water Congress 2005: Impacts of Global Climate Change-Proceedings of the 2005 World Water and Environmental Resources Congress, 2005.
|
| [84] |
Potter BL. Climatic controls on the summertime energy balance of a thermokarst lake in northern Alaska: Short-term, seasonal, and interannual variability[Dissertation]. University of Nebraska, 2011.
|
| [85] |
Robertson E, Barry PJ. The water and energy balances of Perch Lake (1969-1980). Atmosphere-Ocean, 1985, 23(3): 238-253. DOI:10.1080/07055900.1985.9649227 |
| [86] |
Zhang Q, Liu H. Interannual variability in the surface energy budget and evaporation over a large southern inland water in the United States. Journal of Geophysical Research: Atmospheres, 2013, 118(10): 4290-4302. DOI:10.1002/jgrd.50435 |
| [87] |
Xiao W, Zhang Z, Wang W et al. Radiation controls the interannual variability of evaporation of a subtropical lake. Journal of Geophysical Research: Atmospheres, 2020, 125(8): e2019JD031264. DOI:10.1029/2019JD031264 |
| [88] |
Elsawwaf M, Willems P. Analysis of the climate variability on Lake Nasser evaporation based on the Bowen ratio energy budget method. Journal of Environmental Biology, 2012, 33(2): 475-485. |
| [89] |
Wang W, Lee X, Xiao W et al. Global lake evaporation accelerated by changes in surface energy allocation in a warmer climate. Nature Geoscience, 2018, 11(6): 410-414. DOI:10.1038/s41561-018-0114-8 |
| [90] |
Jing SJ, Xiao W, Wang JY et al. Evaporation variability and its control factors of Lake Taihu from 1958 to 2017. J Lake Sci, 2022, 34(5): 1697-1711. [荆思佳, 肖薇, 王晶苑等. 1958—2017年太湖蒸发量年际变化趋势及主控因子. 湖泊科学, 2022, 34(5): 1697-1711. DOI:10.18307/2022.0522] |
| [91] |
Xiao K, Griffis TJ, Baker JM et al. Evaporation from a temperate closed-basin lake and its impact on present, past, and future water level. Journal of Hydrology, 2018, 561: 59-75. DOI:10.1016/j.jhydrol.2018.03.059 |
| [92] |
Mhawej M, Fadel A, Faour G. Evaporation rates in a vital lake: A 34-year assessment for the Karaoun Lake. International Journal of Remote Sensing, 2020, 41(14): 5321-5337. DOI:10.1080/01431161.2020.1739354 |
| [93] |
Min Q, Liu Y. Calculation of lake evaporation and trend analysis of Lake Poyang: 1955-2004. J Lake Sci, 2006, 18(5): 452-457. [闵骞, 刘影. 鄱阳湖水面蒸发量的计算与变化趋势分析(1955—2004年). 湖泊科学, 2006, 18(5): 452-457. DOI:10.18307/2006.0502] |
| [94] |
Shi XH, Li SC, An D et al. A study of the change of Qinghai Lake evaporation. Climatic and Environmental Research, 2010, 15(6): 787-796. [时兴合, 李生辰, 安迪等. 青海湖水面蒸发量变化的研究. 气候与环境研究, 2010, 15(6): 787-796.] |
| [95] |
Huang HJ, Wang YP, Li QH. Evaporation variation from Erhai Lake and its controls under climatic warming. Journal of Meteorology and Environment, 2010, 26(1): 32-35. [黄慧君, 王永平, 李庆红. 气候变暖背景下洱海水面蒸发量的变化及影响因素. 气象与环境学报, 2010, 26(1): 32-35.] |
| [96] |
Zhao X, Liu Y. Variability of surface heat fluxes and its driving forces at different time scales over a large ephemeral lake in China. Journal of Geophysical Research: Atmospheres, 2018, 123(10): 4939-4957. DOI:10.1029/2017jd027437 |
| [97] |
Min Q. Amelioration of wind speed function in Dalton's formula. Hydrology, 2005, 25(1): 37-41. [闵骞. 道尔顿公式风速函数的改进. 水文, 2005, 25(1): 37-41.] |
| [98] |
Riveros-Iregui DA, Lenters JD, Peake CS et al. Evaporation from a shallow, saline lake in the Nebraska Sandhills: Energy balance drivers of seasonal and interannual variability. Journal of Hydrology, 2017, 553: 172-187. DOI:10.1016/j.jhydrol.2017.08.002 |
| [99] |
Lei Y, Yao T, Yang K et al. Thermal regime, energy budget and lake evaporation at Paiku Co, a deep alpine lake in the central Himalayas. Hydrology and Earth System Sciences Discussions, 2020, 1-32. DOI:10.5194/hess-2020-320 |
| [100] |
Lazhu, Yang K, Wang J et al. Quantifying evaporation and its decadal change for Lake Nam Co, central Tibetan Plateau. Journal of Geophysical Research: Atmospheres, 2016, 121(13): 7578-7591. DOI:10.1002/2015jd024523 |
| [101] |
Shao C, Chen J, Chu H et al. Intra-annual and interannual dynamics of evaporation over western Lake Erie. Earth and Space Science, 2020, 7(11): e2020EA001091. DOI:10.1029/2020ea001091 |
| [102] |
Liu H, Blanken PD, Weidinger T et al. Variability in cold front activities modulating cool-season evaporation from a southern inland water in the USA. Environmental Research Letters, 2011, 6(2): 024022. DOI:10.1088/1748-9326/6/2/024022 |
| [103] |
Liu Q, Wang W, Xiao W et al. Quantifying the effects of different cold air events on latent and sensible heat fluxes of Lake Taihu. J Lake Sci, 2019, 31(4): 1144-1156. [刘强, 王伟, 肖薇等. 不同强度冷空气对太湖水热交换的定量影响. 湖泊科学, 2019, 31(4): 1144-1156. DOI:10.18307/2019.0425] |
| [104] |
Fink G, Schmid M, Wahl B et al. Heat flux modifications related to climate-induced warming of large European lakes. Water Resources Research, 2014, 50(3): 2072-2085. DOI:10.1002/2013WR014448 |
| [105] |
Hu C, Wang Y, Wang W et al. Trends in evaporation of a large subtropical lake. Theoretical and Applied Climatology, 2017, 129(1): 159-170. DOI:10.1007/s00704-016-1768-z |
| [106] |
Feng X, Lauder AM, Posmentier ES et al. Evaporation and transport of water isotopologues from Greenland lakes: The lake size effect. Quaternary Science Reviews, 2016, 131: 302-315. DOI:10.1016/j.quascirev.2015.07.029 |
| [107] |
Woolway RI, Jones ID, Maberly SC et al. Diel surface temperature range scales with like size. PLOS ONE, 2016, 11(3): e0152466. DOI:10.1371/journal.pone.0152466 |
| [108] |
Gorham E. Morphometric control of annual heat budgets in temperate lakes. Limnology and Oceanography, 1964, 9(4): 525-529. DOI:10.4319/lo.1964.9.4.0525 |
| [109] |
Dai YJ, Wei N, Huang AN et al. The lake scheme of the Common Land Model and its performance evaluation. Chinese Science Bulletin, 2018, 63(Z2): 3002-3021. [戴永久, 魏楠, 黄安宁等. 通用陆面模式(CoLM)湖泊过程方案与性能评估. 科学通报, 2018, 63(Z2): 3002-3021. DOI:10.1360/N972018-00609] |
| [110] |
Rohwer C. Evaporation from free water surfaces. Technical Bulletin No. 271. Washington, DC: US Department of Agriculture, 1931.
|
| [111] |
Nullet D, Juvik JO. Generalized mountain evaporation profiles for tropical and subtropical latitudes. Singapore Journal of Tropical Geography, 1994, 15(1): 17-24. DOI:10.1111/j.1467-9493.1994.tb00242.x |
| [112] |
Spence C, Rouse WR, Worth D et al. Energy budget processes of a small northern lake. Journal of Hydrometeorology, 2003, 4(4): 694-701. DOI:10.1175/1525-7541(2003)004<0694:ebpoas>2.0.co;2 |
| [113] |
Rouse WR, Blanken PD, Bussieres N et al. An investigation of the thermal and energy balance regimes of Great Slave and Great Bear Lakes. Journal of Hydrometeorology, 2008, 9(6): 1318-1333. DOI:10.1175/2008jhm977.1 |
| [114] |
Finch JW. A comparison between measured and modelled open water evaporation from a reservoir in south-east England. Hydrological Processes, 2001, 15(14): 2771-2778. DOI:10.1002/hyp.267 |
| [115] |
Sturrock AM, Winter TC, Rosenberry DO. Energy budget evaporation from Williams Lake: A closed lake in north central Minnesota. Water Resources Research, 1992, 28(6): 1605-1617. DOI:10.1029/92WR00553 |
| [116] |
Cui L, Mu ZX, Chen P et al. Analysis of evaporation from Ebinur Lake. Water Resources Protection, 2012, 28(6): 59-61. [崔龙, 穆振侠, 陈平等. 艾比湖湖水蒸发量分析. 水资源保护, 2012, 28(6): 59-61.] |
| [117] |
Rosenberry DO, Winter TC, Buso DC et al. Comparison of 15 evaporation methods applied to a small mountain lake in the northeastern USA. Journal of Hydrology, 2007, 340(3): 149-166. DOI:10.1016/j.jhydrol.2007.03.018 |
| [118] |
Bouin MN, Caniaux G, Traulle O et al. Long-term heat exchanges over a Mediterranean lagoon. Journal of Geophysical Research: Atmospheres, 2012, 117: D23104. DOI:10.1029/2012jd017857 |
| [119] |
Dutton JA, Bryson RA. Heat flux in Lake Mendota. Limnology and Oceanography, 1962, 7: 80-97. DOI:10.4319/lo.1962.7.1.0080 |
| [120] |
Shao C, Chen J, Stepien CA et al. Diurnal to annual changes in latent, sensible heat, and CO2 fluxes over a Laurentian Great Lake: A case study in Western Lake Erie. Journal of Geophysical Research: Biogeosciences, 2015, 120(8): 1587-1604. DOI:10.1002/2015JG003025 |
| [121] |
Gianniou SK, Antonopoulos VZ. Evaporation and energy budget in Lake Vegoritis, Greece. Journal of Hydrology, 2007, 345(3): 212-223. DOI:10.1016/j.jhydrol.2007.08.007 |
| [122] |
Giadrossich F, Niedda M, Cohen D et al. Evaporation in a Mediterranean environment by energy budget and Penman methods, Lake Baratz, Sardinia, Italy. Hydrology and Earth System Sciences, 2015, 19(5): 2451-2468. DOI:10.5194/hess-19-2451-2015 |
| [123] |
Yamamoto G, Kondo J. Evaporation from Lake Towada. Journal of the Meteorological Society of Japan, 1964, 42(2): 85-96. |
| [124] |
Sun J, Hu W, Wang NA et al. Eddy covariance measurements of water vapor and energy flux over a lake in the Badain Jaran Desert, China. Journal of Arid Land, 2018, 10(4): 517-533. DOI:10.1007/s40333-018-0057-3 |
| [125] |
Han PF, Wang XS, Hu XN et al. Dynamic relationship between lake surface evaporation and meteorological factors in the Badain Jaran Desert. Arid Zone Research, 2018, 35(5): 1012-1020. [韩鹏飞, 王旭升, 胡晓农等. 巴丹吉林沙漠湖泊水面蒸发与气象要素的动态关系. 干旱区研究, 2018, 35(5): 1012-1020. DOI:10.13866/j.azr.2018.05.02] |
| [126] |
Aydin H, Karakuş H. Estimation of evaporation for Lake Van. Environmental Earth Sciences, 2016, 75(18): 1275. DOI:10.1007/s12665-016-6077-4 |
| [127] |
Martínez-Alvarez V, Gallego-Elvira B, Maestre-Valero JF et al. Simultaneous solution for water, heat and salt balances in a Mediterranean coastal lagoon (Mar Menor, Spain). Estuarine, Coastal and Shelf Science, 2011, 91(2): 250-261. DOI:10.1016/j.ecss.2010.10.030 |
| [128] |
Gallego-Elvira B, Baille A, Martín-Górriz B et al. Energy balance and evaporation loss of an agricultural reservoir in a semi-arid climate (south-eastern Spain). Hydrological Processes, 2010, 24(6): 758-766. DOI:10.1002/hyp.7520 |
| [129] |
Li WC, Li SJ, Pu PM. Estimates of plateau lake evaporation: A case study of Zige Tangco. J Lake Sci, 2001, 13(3): 227-232. [李万春, 李世杰, 濮培民. 高原咸水湖水面蒸发估算——以兹格塘错为例. 湖泊科学, 2001, 13(3): 227-232. DOI:10.18307/20010305] |
| [130] |
Guo Y, Zhang Y, Ma N et al. Long-term changes in evaporation over Siling Co Lake on the Tibetan Plateau and its impact on recent rapid lake expansion. Atmospheric Research, 2019, 216: 141-150. DOI:10.1016/j.atmosres.2018.10.006 |
| [131] |
Yu S, Liu J, Xu J et al. Evaporation and energy balance estimates over a large inland lake in the Tibet-Himalaya. Environmental Earth Sciences, 2011, 64(4): 1169-1176. DOI:10.1007/s12665-011-0933-z |
| [132] |
Abtew W. Evaporation estimation for Lake Okeechobee in South Florida. Journal of Irrigation and Drainage Engineering, 2001, 127(3): 140-147. DOI:10.1061/(ASCE)0733-9437(2001)127:3(140) |
| [133] |
Ali S, Ghosh NC, Singh R. Evaluating best evaporation estimate model for water surface evaporation in semi-arid region, India. Hydrological Processes, 2008, 22(8): 1093-1106. DOI:10.1002/hyp.6664 |
| [134] |
Lewis Jr. WM. Temperature, heat, and mixing in Lake Valencia, Venezuela. Limnology and Oceanography, 1983, 28(2): 273-286. DOI:10.4319/lo.1983.28.2.0273 |
| [135] |
Vallet-Coulomb C, Legesse D, Gasse F et al. Lake evaporation estimates in tropical Africa (Lake Ziway, Ethiopia). Journal of Hydrology, 2001, 245(1): 1-18. DOI:10.1016/S0022-1694(01)00341-9 |
| [136] |
Yin X, Nicholson SE. The water balance of Lake Victoria. Hydrological Sciences Journal, 1998, 43(5): 789-811. DOI:10.1080/02626669809492173 |
| [137] |
Verburg P, Antenucci JP. Persistent unstable atmospheric boundary layer enhances sensible and latent heat loss in a tropical great lake: Lake Tanganyika. Journal of Geophysical Research: Atmospheres, 2010, 115(D11): D11109. DOI:10.1029/2009JD012839 |
| [138] |
Delclaux F, Coudrain A, Condom T. Evaporation estimation on Lake Titicaca: A synthesis review and modelling. Hydrological Processes, 2007, 21(13): 1664-1677. DOI:10.1002/hyp.6360 |
| [139] |
McJannet D, Cook F, McGloin R et al. Long-term energy flux measurements over an irrigation water storage using scintillometry. Agricultural and Forest Meteorology, 2013, 168: 93-107. DOI:10.1016/j.agrformet.2012.08.013 |
| [140] |
Alfonso MB, Brendel AS, Vitale AJ et al. Impact of heatwave events on latent and sensible surface heat flux and future perspectives in shallow lakes based on climate change models. Cuadernos de Investigación Geográfica, 2021, 47(2): 19. DOI:10.18172/cig.4456 |
 2023, Vol. 35
2023, Vol. 35